Grade Adjusted Pace
The concept of Grade Adjusted Pace was popularised by Strava. The principle is to work out the equivalent pace on level ground for running uphill or downhill. Equivalence here is generally based on equivalent energy demand. Obviously, running uphill or downhill at the same effort has differences in which muscles are used, your biomechanics, and the degree of delayed onset muscle soreness. However, for most purposes, your Grade Adjusted Pace is a lot more useful than your unadjusted pace.
Contents
1 Uses of Grade Adjusted Pace
You can use great adjusted pace to calculate a grade adjusted distance. If you are managing your training using volume metrics, and you run in hilly terrain, then great adjusted distance is a Far more valid metric than unadjusted distance. If you manage your training with intensity, then knowing your Grade Adjusted Pace will give you a better measure of intensity. (using running power is even better, such as Stryd.)
2 The formulas
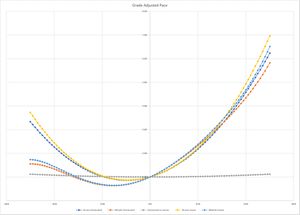
Several research studies have looked at the energy cost of running on inclined treadmills to create formulas for grade adjusted pace. The studies have the advantage of laboratory grade gear to accurately measure energy usage. The big downside is the small sample size. Strava have refined these formulas based on millions of runs from hundreds of thousands of athletes. This big data approach produces a formula with wider validity.
2.1 The Minetti Formula
This is the original study used by Strava before their big data refinement was Minetti:
155.4i5 - 30.4i4 - 43.3i3 +46.3i2 - 165i + 3.6
Where i is the grade, with 0.01 is 1% grade. You can divide by 3.6 to get the relative cost. Note that the treadmill speed used in the study is the speed moved. If you are looking at horizontal speed from GPS data, then you need to use Pythagoras to calculate the actual speed. This becomes significant on steep hills.
3 The Strava Formula
The Stava formula isn't published, but it is roughly:
15.14i2 - 2.896i
Unlike Minetti, the formula gives the relative cost, not the absolute cost.
3.1 Comparison
4 Stryd
Stryd gives very few details on their adjustment for their grade adjustment. The only blog post from 2019 gives a very simplistic linear formula that's obviously wrong. In my experience, it seems that Stryd underestimates power on downhill running.